Next: Shift-and-Invert QEP.
Up: Spectral Transformations for QEP
Previous: Invert QEP.
Contents
Index
With a shift
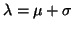 ,
the shifted QEP is
,
the shifted QEP is
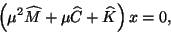 |
(260) |
where 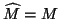 ,
,
 ,
and
,
and
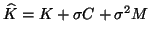 .
The shift transforms eigenvalues
.
The shift transforms eigenvalues  of (9.1) close to
of (9.1) close to
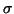 into eigenvalues
into eigenvalues 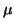 close to
close to  .
.
The corresponding generalized ``linear'' eigenvalue problem is (again
in terms of  , rather than
, rather than 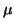 )
)
or
if the Hermitian of the matrix triplet 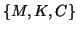 wants to be preserved.
wants to be preserved.
Susan Blackford
2000-11-20
![]() , rather than
, rather than ![]() )
)