




Next: Example 8.6.3.
Up: Numerical Examples
Previous: Example 8.6.1.
Contents
Index
Even when the initial vector is premultiplied by 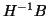 , the
Ritz vectors may be further improved by implementing the postprocessing step
shown in (8.27). The following example represents the
same shaft as in Example 8.6.1, but with a coarser grid so that the order of
, the
Ritz vectors may be further improved by implementing the postprocessing step
shown in (8.27). The following example represents the
same shaft as in Example 8.6.1, but with a coarser grid so that the order of
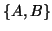 is 160. A shift of
is 160. A shift of 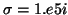 was used and full
orthogonality was
maintained. The following table shows that the postprocessing step improves
the direct residuals by up to four orders of magnitude.
was used and full
orthogonality was
maintained. The following table shows that the postprocessing step improves
the direct residuals by up to four orders of magnitude.
| |
|
|
| Ritz value |
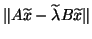 |
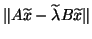 |
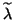 |
(with postprocessing) |
(no postprocessing) |
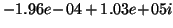 |
 |
 |
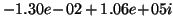 |
 |
 |
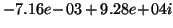 |
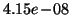 |
 |
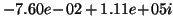 |
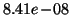 |
 |
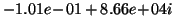 |
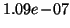 |
 |
Susan Blackford
2000-11-20