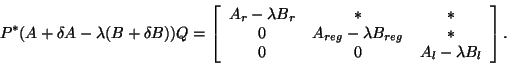Next: Software Availability
Up: GUPTRI Algorithm
Previous: Criterion for Determining the
Contents
Index
The GUPTRI Form of a Regularized Problem.
The deflations made in the staircase
algorithm result in an exact GUPTRI form of a nearby matrix
pencil
 :
:
If all range/null space separations
in the GUPTRI algorithm are well defined with respect
to the deflation tolerance parameters 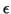 and gap, then
and gap, then
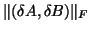
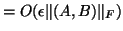 .
The nearby problem represents a regularized problem that
has a stable Kronecker structure with respect to the
deflation criteria of the algorithm.
.
The nearby problem represents a regularized problem that
has a stable Kronecker structure with respect to the
deflation criteria of the algorithm.
Susan Blackford
2000-11-20
![]() :
: