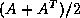 of the coefficient matrix
of the coefficient matrix 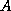 leads to a method that does not need this extended storage.
Such a method was proposed by Concus and Golub [56]
and Widlund [216].
leads to a method that does not need this extended storage.
Such a method was proposed by Concus and Golub [56]
and Widlund [216].
In §![]() we pointed out that conjugate
gradient methods for non-selfadjoint systems require the storage of
previously calculated vectors. Therefore it is somewhat remarkable
that preconditioning by the symmetric part
we pointed out that conjugate
gradient methods for non-selfadjoint systems require the storage of
previously calculated vectors. Therefore it is somewhat remarkable
that preconditioning by the symmetric part 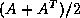 of the coefficient matrix
of the coefficient matrix 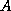 leads to a method that does not need this extended storage.
Such a method was proposed by Concus and Golub [56]
and Widlund [216].
leads to a method that does not need this extended storage.
Such a method was proposed by Concus and Golub [56]
and Widlund [216].
However, solving a system with the symmetric part of a matrix may be no easier than solving a system with the full matrix. This problem may be tackled by imposing a nested iterative method, where a preconditioner based on the symmetric part is used. Vassilevski [212] proved that the efficiency of this preconditioner for the symmetric part carries over to the outer method.